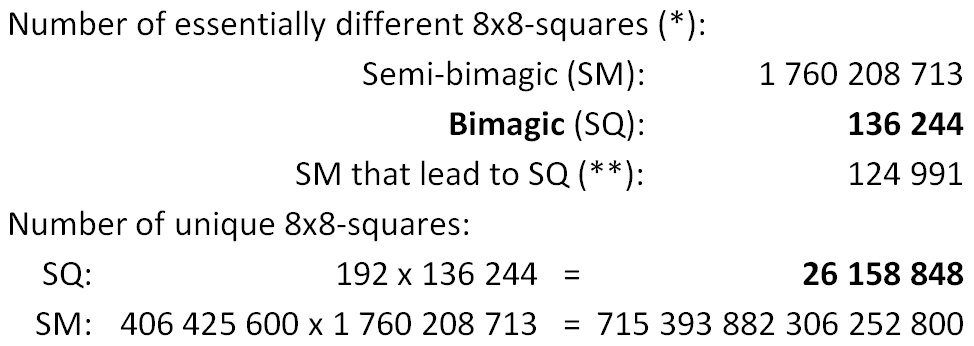
All bimagic squares of order 8

Total Number

= 192 · 136 244 =

26 158 848

Abbreviations: SQ = bimagic square of order 8 SM = semi-bimagic square of order 8

The complete sets of all SM and SQ were found by Walter Trump and Francis Gaspalou in April 2014.

Common paper of Walter Trump and Francis Gaspalou about the calculation of all bimagic 8x8 squares (PDF)

(*) Beside rotations and reflections there are 192 permutations of rows and columns under which all magic 8x8-squares are invariant.
For SM the number of permutations is (8!/2)².
(**) By permutations of rows and columns a given SM can be transformed (or not) into one or several SQ. See PDF: SQ from same SM

Methods for the calculation of SM

Read how semi-magic 8x8-squares can be described by 16 integers.

Transformations and standard positions

Transformations that work on bimagic 8x8 squares.

Interesting subsets of SQ with certain properties

See some special SQ and download different subsets.

Confirmations of the results

Read what confirmations are already done and which should be done next.

Distribution of SM and SQ

Dwane Campbell could solve the hot spot problem.
But there are still some open questions about the strange distribution of the squares.

Bimagic Queen's tours

NEW: first bimagic queen's tours

William Walkington found the first bimagic queen's tour on 2020-07-23.
Within our set of semi-bimagic 8x8-squares further queen's tours could be found in August 2020.
Notes about other chess tours
- PDF: Bimagic or semi-bimagic rook's, king's and knight's tours
- PDF: Incomplete diagonally bimagic chess tours on an 8x8-board
- PDF: Diagonally bimagic queen's tours on larger boards

Most important data for download

- ZIP: Complete list of all essentially different SQ (9 MB)
- ZIP: List of all SM that lead to SQ (8 MB)
The second number indicates how many ess. diff. SQ can be created from a certain SM.

Research on bimagic 8x8-squares done by Francis Gaspalou before

- PDF: How many squares are there, Mr. Tarry?
- PDF: Revisit of the method of construction of the first bimagic squares
- PDF: About the Rilly's method for generating 8x8 bimagic squares
- PDF: Derived Methods from those of Coccoz and Rilly

What about calculating all bimagic 9x9-squares?

In 2005 I estimated the number of bimagic squares by stochastical methods (estimates of multimagic squares).
The number of bimagic 8x8-squares was estimated to 2×10⁰⁷. Now we know the correct number: 2.6×10⁰⁷. That is a little greater, because of weak symmetry advantages.
We expect about 10²² bimagic squares of order 9. That is so very very much more. A calculation is definitively not possible with our methods.
But it should be possible to determine certain subsets, like the centrally symmetrical (= associative) bimagic squares of order 9.

Home: Notes on Magic Squares